Numerical analysis
(Francesco Ballarin, Maurizio Paolini)
One of the task of the numerical analysis is to find the approximate solutions of some complex problems for which an exact solution cannot be found analytically. Such problems often involve partial differential equations and typically emerge from concrete situations (for example in physics, engineering and finance) or from a purely theoretical-mathematical context. The approximate solutions are obtained by means of computers, and for this reason there is a natural interdisciplinary connection with computer science which is also present in the Department.
1. Geometric evolution of surfaces
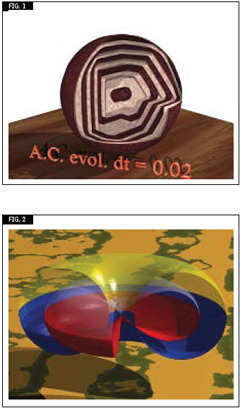
In this framework, the group works on the numerical simulation of the evolutions of fronts. The paradigmatic model is the evolution of surfaces in the three dimensional space (or ipersurfaces in high dimensional spaces) with a velocity proportional to the average local curvature. In Figure 1 for example is shown a three dimensional section of a torus immersed in the four dimensional space that evolves in time with the law mentioned before. In Figure 1 the ipersurface is shown at two different times: one of this is the time at which the hole of the torus closes and the surface changes its topology. In Figure 2 is shown the result of the evolution of a surface (spherical at the beginning) under a similar kind of law but in a anisotropic environment (crystalline anisotropy in this case).
2. Computational fluid dynamics and computational mechanics
The problems of interest, modelled by partial differential equations, are often derived from mathematical physics models, for instance in solid mechanics, fluid dynamics and porous media flow. In this context, the group works on the formulation and on the analysis of numerical analysis techniques capable of solving such problems, and especially techinques based on finite element methods.
Research efforts are devoted to methodological aspects, as well as concrete applications. Indeed, concerning research topics on the formulation of numerical methods, the main goals we pursue are within the study of finite element methods, and their variants. Such methods are afterwards applied to real-life problems, such as computational fluid dynamics for the human vascular system or flow in porous media.
3. Reduced order modelling
Numerical simulations of problems governed by partial differential equations often requires very large computational efforts, especially for evolution problems (such as those in the first research line) or complex problems (such as those in the second research line). Large computational times are only compatible with one, or a handful, of simulations of the problem of interest, but rapidly become unaffordable when the interest is solving inverse problems, such as the optimization of a parameter on which the problem of interest depends. For this reason, the group develops several reduced order modelling techniques, and in particular reduced basis methods. Those methods allow to combine the methodological rigor of finite element methos (for instance, availability of error estimates) with considerably lower required computational time (even up to five orders of magnitude, in the best cases), without sacrifing the accuracy of the solution.
4. Open-source software development for teaching and research
The group develops open-source software for research purposes within the above research lines, but also for teaching purposes. Among the most recent projects, we mention:
- RBniCS - reduced order modelling in FEniCS, mainly developed within the third research line,
- multiphenics/multiphenicsx - easy prototyping of multiphysics problems in FEniCS/FEniCSx, mainly developed within the second research line,
- FEMlium - interactive visualization of finite element simulations on geographic maps with folium, mainly developed within the second research line,
- FEM on Colab/FEM on Kaggle, mainly developed for teaching purposes.
Collaborations
- Cornell University (United States)
- Florida State University (United States)
- Hokkaido University (Japan)
- Politecnico di Milano (Italy)
- Sandia National Laboratories (United States)
- Scuola Internazionale Superiore di Studi Avanzati (Italy)
- Università di Pisa (Italy)
- Università di Roma Tor Vergata (Italy)
- University of Warsaw (Poland)
- Virginia Tech (United States)